A grade 5 teacher approached me asking if we could plan for a lesson toward the end of her unit on multiplication. To start, we grabbed our curriculum to see what the specific expectations told us. Specifically, she told me that she had been trying to help her students reach this expectation:
-Multiply two-digit whole numbers by two-digit whole numbers, using estimation, student-generated algorithms, and standard algorithms
I asked her what her students had done so far and was given a list of experiences including working with manipulatives, word problems and time to practice… I asked her how her students were doing so far, and was told that her students were doing very well, with a few exceptions (4 boys and 1 girl were still struggling a bit). I then asked her to look at the curriculum expectation with me again, pointing out a piece I wanted us to look at deeper:
-Multiply two-digit whole numbers by two-digit whole numbers, using estimation, student-generated algorithms, and standard algorithms
I asked which of the three (estimation, student generated algorithms, and standard algorithms) she had spent the most time on… to which I was met with a stunned look. She shared with me that all of her time had been spent trying to help her students be able to understand the standard algorithm, but she felt that her students were doing really well anyway.
I asked if we could try something a little different, and see how well her students could estimate, to which she was eagerly agreeable. Together we came up with this problem: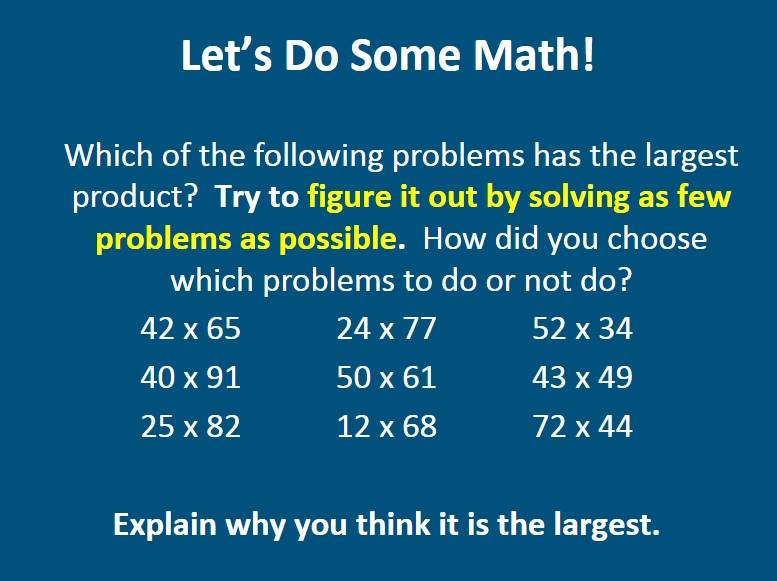
Before we started the lesson, I asked her how she would go about completing this task. She pointed to the pieces in the picture that she could eliminate and gave clear explanations as to why they were definitely smaller than others (i.e., 42 x 65 must be smaller than 40 x 91 because there are a lot more 40s…).
As we walked into class she turned to me and pointed out the 5 students (4 boys and 1 girl) she believed would really struggle with the task. Then, together we introduced the task and explained to her students that they had to do as few as possible to show us which one had the largest product, then be able to prove to the class how they knew it was the largest. Students were then given time to think and work and show their reasoning.
The result of this problem couldn’t have been more revealing than it was! Almost every student silently worked on and answered EVERY ONE of the given problems… all except for 6 students… 5 of whom were the students the teacher had just identified as struggling students! These 6 had eliminated problems they realized they didn’t need to do, only working out those they weren’t sure about. Only these 6 did any estimating at all!!!
We gave time for each of the 6 to explain how they started eliminating questions that they knew they didn’t have to do, and the rest of the class were quite receptive to their thinking/reasoning. The teacher and I drew open arrays that were approximately proportional for us to think about the dimensions involved to represent what the 6 students had been explaining.
In the end, we gave a quick exit card with a few questions again asking which was the largest, to work out as few as possible, and asked for their reasoning… but still many struggled with this and just did them all.
After being part of this lesson I couldn’t help but reflect on a few really big questions:
- I wonder what it means to be good at multiplication?
- I wonder what it means to be good at math?
- I wonder if many other students in other classrooms struggle specifically with the procedures, yet are capable of thinking mathematically?
- I wonder which is better:
- A student who can dutifully enact the steps of an algorithm quickly and accurately, yet can’t estimate, visualize, conceptualize what the algorithm is doing… or
- A student who sometimes struggles with the algorithm, yet has a strong grasp of what multiplication means?
- I wonder WHY students who were SO successful with their learning multiplication so far struggled with this problem?
- I wonder what our students think being successful in mathematics means?
- I wonder what messages we send to make them believe this???
I’m also left with a thought about the curriculum and how we perceive what it is asking us to do. I believe that when we read our curriculum, we read it through the lens of what we value, what we understand, and our own personal experiences! And for many of us, what we end up valuing is the skill… or the procedure… or the knowledge… Yet, for me, I wish we also valued understanding… and thinking… and reasoning…
Great article, Mark! It seems like for many the standard algorithm is the goal of a unit like this and that all the other “stuff” is there to support that goal. Being good at math means being able to recall facts quickly and complete the steps accurately. One thing I’ve been communicating w/ teachers is that, while algorithms are a valid and widely used mathematical tool, they represent the end of a learning progression. On the other hand, strategies and algorithms based on flexible thinking, place value, and the properties of the operations help w/ calculating while at the same time prepare students for higher levels of maths down the road.
LikeLike
Hi Chris. Yet those who could do what you say is the “end of a learning progression” (they could multiply accurately) could not estimate? They could not visualize how big the numbers would be? The concept of multiplication wasn’t there… but the procedures were.
I wonder what happens if we skip the progression itself and aim for the end?
I also wonder if estimation important? According to your list, all that matters is that they can get the answer quickly.
LikeLike
this awareness of what i call concept vs bookkeeping i use in giving feedback to students on their work … it seems to work well with comm. coll. students … it helps clarify that generally they know more than they thought.
LikeLiked by 1 person
Hi Mark,
Your blog post really resonates with me. And my initial reactions are that many elementary teachers don’t know the reasoning, the thinking, and the understanding behind some of the bigger concepts. They head to the algorithm because it’s the short cut. Math is the uncomfortable subject for them and they fear it.
I would have tried an Openmiddle.com type question…using the numbers 1-9, which 2 digit number multiplied by a 2 digit number result in the greatest product.
Thanks for your thoughts.
Kristen
LikeLike
That would be a great way to help our students understand what happens to the product based on place value. Also think somehow students need to visualize the open arrays for these too.
LikeLike
Love the task! Thank you so much for sharing. I’m curious to try it with both preservice and practicing teachers, as well as with students. I began considering dimensions of the rectangles and maximizing the area, as well as just what I notice about combining groups. This task takes us all so much farther toward the depth and rigor of understanding we want of all of our students. In addition, as you imply, those students who may not have been as adept at the standard algorithm, might indeed be our best mathematical thinkers as they go beyond the surface expectations to examine and consider mathematical relationships and magnitudes.
Thanks for making me think!
DeAnn
LikeLiked by 1 person
for deann … wrote this up a while back …
Maximum Area with Fixed Perimeter
Introduction
In Lancelot Hogben’s 1960 Mathematics in the Making (page 214), he discusses the relationship between a parabola and the area of a rectangle. This isn’t a secret; it simply isn’t talked about very much in class. However, I believe it should be discussed because it’s one of those “oh, really?” math relationships that captures students’ attention.
The Story
The question is: what is the maximum area of a rectangle with a fixed perimeter?
For example, if the perimeter of a rectangle is fixed at 20 units, how many configurations of a rectangle are there and which one gives the greatest area?
Given this, start with a length of 1 with the corresponding width of 9, and the area is therefore 9. Yes, I know – length is typically longer than width but for purposes of this discussion, allow me to breach this definition. Building a table would give:
L W Area
1 9 9
2 8 16
3 7 21
4 6 24
5 5 25
6 4 24
7 3 21
8 2 16
9 1 9
In this case, as will happen in every case, the maximum area will occur when there is a square, and the general formula is that the maximum area (MA) = (P/4)2, where P is the perimeter.
On further examination, if the points in the above table were plotted with width (or length) as the independent variable and area as the dependent variable, the outcome would be a parabola with vertex at (5,25).
With a vertex = (5,25) and point (2, 16), the equation for the parabola can be derived and gives the equation of this parabola as y = 10x – x2, which is exactly the same as x(10 – x) for calculating area from P = L•W.
No surprise here but the point is that this relationship exists and is typically not ever noted in the classroom when addressing either of the traditional formulae for linear or quadratic equations.
LikeLike