A few years ago I was teaching a 6/7 split class. We had been exploring surface area and volume of prisms (rectangular so far), but were quite early on in the learning cycle. At the beginning of my math class I drew a picture of a rectangular prism and wrote the dimensions on the diagram like below:

I asked everyone to calculate the Surface Area. Really this was just a check for me to see if we understood surface area… the problem for the day was coming up.
However, as I walked around, I realized something VERY unusual. EVERYONE got the answer of 250… but not everyone did it correctly. Let me show you:

Take a look at the 2 answers above. Some students calculated the Surface Area correctly at 250 units squared… and others calculated 250 units cubed (they found the volume).
I apologized to the class for giving them the worst problem ever… THE ONLY POSSIBLE rectangular prism that has the same surface area as volume. Then one student commented, “Is that the only possible prism?”
I didn’t know the answer, so we started seeing if it was the only possible prism.
Over the next 100 minutes, every student in the class, some in pairs, some on their own, started drawing prisms and calculating the surface area and volume.
Eventually, a student told me that one of the dimensions couldn’t possibly be a 1. I asked him to prove it… After a few minutes he showed me several examples with numbers that were small or large. He attempted to find the limits:
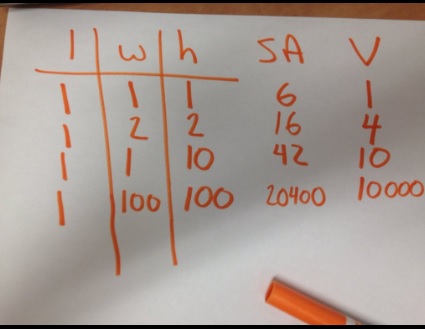
He explained to the class that if there is a 1 as any of the dimensions, the Surface Area would always be larger. We explored why that happens.
Minutes later, another student told me that it was impossible to have a 2 as one of the dimensions. I asked her to prove it… She showed me the work that she had completed and shared it with the class.

She explained that the numbers were getting close if she chose 2 of the numbers identical, but the end pieces of the shape would always add up to more than the volume would.
After lots of trial and error… guessing and refining ideas, several students started finding possible prisms that also had the same Surface Area and Volume…
The class started noticing a pattern between the dimensions and found limits between the smallest and largest possible prisms…
By the end of class, all students had calculated dozens of surface areas and volumes… all students were making conjectures or testing out the conjectures of others.
My original problem, which I intended as a quick warm-up was not a quality engaging problem. However, I want you to think about what made this lesson better?
WHO posed the problem that day? Did this have something to do with the shared responsibilities that happened later in the lesson?
What if I just moved on? would the learning have been as rich?
Think about how the students picked the shapes they were testing? Some students worked in teams to work strategically… others made their own conjectures and followed those patterns.
In this lesson, choice was key, but the choices didn’t come from me… Students were working together to reach an ultimate goal, not in competition with each other… Conjectures were made and tested, not because I told everyone to, but because it served our purpose!
I think about Dan Meyer’s “Real World vs Real Work” a lot. Why were my students so engaged here? There was NO real world connection. That wasn’t what was motivating my students at all!

I also think we need to reflect on the level of cognitive demand we ask our students to be engaged in:

“Doing Mathematics Tasks” seems like something that is hard to do, yet, every one of my students were engaged in this problem… everyone eagerly searched for patterns, many drew pictures, used snap cubes, visualized what was happening… all in the name of better understanding the relationships between the dimensions of a rectangular prism, and its surface area and volume!
By the end of the class, my students had found exactly 10 rectangular prisms that have the same value of its surface area as its volume (using only whole numbers), and could prove that these were the only 10 possible.
I’d love to hear your thoughts about our problem… or why the students were SO engaged… or about the conditions that must have been present in the class… or how problem solving can be used as a purposeful practice of procedures…..
Self-determination is a big part of it isn’t it. That you’d apologised for the task made the question that followed even more the students’. Also, the open-ended nature of it. There wasn’t just one answer to find, and then everyone hears through the grapevine that a fast student has found it.
LikeLiked by 1 person
I love that you finally published this lesson. I think of it often.
I’ll have more to say soon but after a lesson today, I have to note how helpful tables are when we need to organize our thinking. A tool our students need to be introduced to and have experiences needing. Love it! We’ve been using ratio tables for Fostnot”s Best Buys unit with crazy “ahha” moments.
LikeLike
You have hit upon the power of emergent inquiry for student engagement. Student raised questions with teachers in co-learner mode. The excitement of seeing ‘what’ with ‘why’! Note too, the flexibility you had to run for 100 min. No, “sorry, we will break for band now”. As a secondary teacher, the richness I had with pod teaching math & science to the same students was the added flex to go longer on emergent inquiries and to ride the student owned pursuits to a fuller understanding. You can now use the same ‘worst problem’ with another class but introducing it as a the story of the old class and the point they raised that you didn’t know the answer to.
I would ensure not to use the language “the same surface area as volume” but to use the same numerical value. 6 mice is not the same as 6 elephants just the same quantity of different things.
Thanks for another nice investigation story for my classes.
LikeLiked by 1 person
Curiosity is a powerful motivator – for many reasons, this sparked curiosity and a need to just know….one exploration led to another…and as so often happens when people explore their own questions, the learning is rich and the journey is the point, with many “sights”explored along the way. Striving for more days that unfold as you described. Thank you for sharing the experience. It sparked many ideas for me.
LikeLiked by 1 person
it’s not the problem that generated the students engagement … it’s that they were allowed by you to engage their imaginations and play with an idea …
LikeLiked by 1 person
Thanks for the comment Mark! While I do think that the notion of finding relationships between concepts, particularly with measurement concepts valuable, I think you are right that the success of the lesson came down to the students being invested in, or “playing”, as you said, with the math.
LikeLike
superb example of inquiry based learning-thanks for sharing johnny israel
LikeLiked by 1 person
It seems to me that there is another ingredient here that turned the “worst problem ever” into a rich investigation–and that is that the “worst problem” was introduced into a classroom environment where the students were comfortable posing questions and encouraged to explore. Without a classroom environment where students feel safe asking questions and taking risks such an apology would have been followed by………crickets…..nothing….nada.
LikeLiked by 1 person
I love that your “worst problem” actually turned into a great formative assessment for you and led to a great discussion with your students.
LikeLiked by 1 person
I think we as teachers are also always learning. While teaching my first year of math to grade 7s. I too at times created “the worst math problem ever” — I did not have the confidence to “let the students play” to determine why the problem “didn’t fit” or was “bad” . I think you allowing the students to explore is testament to your confidence in the classroom and your flexibility. I am always so concerned with covering the material that I find myself “shutting down” the inquiry when it naturally arises. Lesson for me to just ‘go with it” there are so many rich and meaningful learning opportunities that are not scripted nor planned!
LikeLike
I had an experience years ago where a short lab (comparing height to arm span) turned into a student inquiry project. At some point, as we were graphing our data, someone wondered if kids at different ages would have the same correlation as adults. So, we measured 4-6 students from each grade K – 12 and the faculty. The kids were super engaged and really invested in the data collection and analysis. (Being at a private school with a shared campus for pre-K – 12th grade made this a lot easier.)
When I tried to replicate the project the next year with a different class, it fell a bit flat. Having me ask the question made it work. When they ask the question, then it is choice, voice, and investigation. The struggle is to create opportunities for them to notice and wonder about things that lead to these rich experiences.
LikeLike
Very interesting post. Nice that it turned into a learning situation for all. I’ve been doing some research to try to find out more about the additional prism’s dimensions for which the same holds true along with a proof, but haven’t had any luck. Can you provide them or direct me to a source where I can find more information.
Best,
Cindy
LikeLike
My students attempted to find limits. Have you found any of them yet?
LikeLike
Haven’t had time to work on it yet.
LikeLike
Just wondering how much the difference between units squared and units cubed came up in your discussions? This is something my students and I have discussed a lot when I too have created problems where, for example, the perimeter and area of a figure are the same number but different units…making them very different measures.
LikeLike
Very inspirational- Thank you!
LikeLike
This is a CLASSIC that should not be lost on the internet.
Who’s collecting problems like this? They should be compiled and sorted by concept and very broad grade bands.
LikeLike
Classrooms are complicated, and many elements contributed to the success of this lesson. Classroom culture: students trusted themselves to explore. Availability of tools: they knew to set up tables for their investigation. A flexible teacher who was willing to seize the opportunity, and to postpone the discussion units. All those things played a part, but do not overlook the quality of the problem itself. It was readily understandable, neither too hard nor too easy, and lent itself to trial and error. There are plenty of questions a student could have asked which would not have yielded as good a lesson, or even anything at all, but this student (and this teacher!) hit the jackpot.
One replicable feature of this problem is that it reverses a routine exercise. That is a reliable way to make things interesting. Find an equation whose solution is x=5. Find fractions whose sum is 3/4. Find a quadratic function with intercepts (1,0), (2,0) and (0,6). And so on. Whenever teaching anything, I ask myself whether there’s a good way to reverse the standard exercise.
LikeLike
There are other rectangular prisms where SA = V. Try 10, 10, 10/3
LikeLike
Ah yes, there are likely an infinite number of prisms. Thank you for finding one of these I was unaware of. However, on direction from my students, they were looking for prisms that have dimensions of positive integers.
LikeLike
The original post included:
“…THE ONLY POSSIBLE rectangular prism that has the same surface area as volume.”
Any w, h, and l that satisfy
(1/w) + (1/h) + (1/l) = .5
will work. You can easily see from this equation why none of the dimensions can be 1 or 2.
Another solution is 3, 12, 12.
LikeLike
A successful lesson is not about using a rich activity, but about using an activity richly. This is what you have done – you created opportunities for students to think mathematically. Thank you for sharing your students’ experience.
LikeLike
What a great story! I am sure you would have had just as much engagement asking for integer solutions to lwh = 2(lw + lh+ wh)! Did you try replicating the experience with another group of students in the intervening years? How much of the success is serendipity and how much is replicable, do you think?
LikeLike