What Makes Math Interesting Anyway?

Many teachers are comfortable allowing their students to read for pleasure at school and encourage reading at home for pleasure too. Writing is often seen as a creative activity. Our society appreciates Literacy as having both creative and purposeful aspects. Yet mathematics as a source of enjoyment or creativity is often not considered by many. 

I want you to reflect on your own thinking here. How important do you see creativity in mathematics? What does creativity in mathematics even mean to you?

Marian Small might explain the notion of creativity in mathematics best. Take a look:

Marian Small – Creativity and Mathematically Interesting Problems from Professional Learning Supports on Vimeo.

Type 1 and Type 2 Questions

Several years ago, Marian Small tried to help us as math teachers see what it means to think and be creative in mathematics by sharing 2 different ways for our students to experience the same content. She called them “type 1” and “type 2” questions.

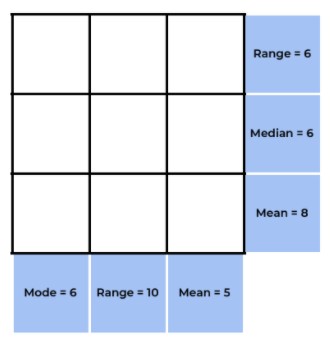
Type 1 problems typically ask students to give us the answer.  There might be several different strategies used… There might be many steps or parts to the problem.  Pretty much every Textbook problem would fit under Type 1.  Every standardized test question would fit here.  Many “problem solving” type questions might fit here too.

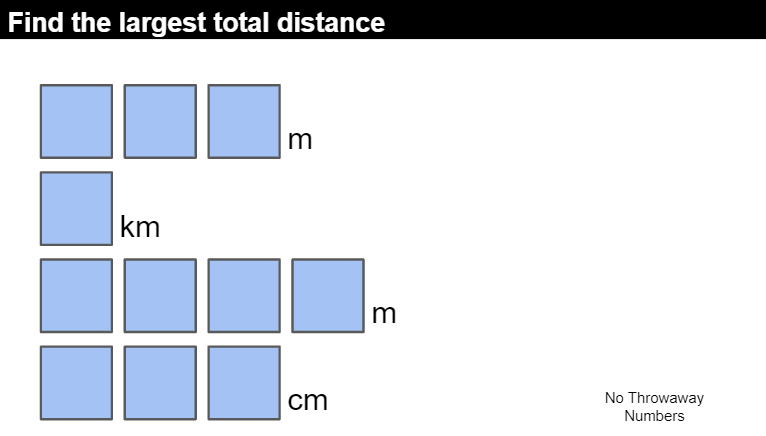
Type 2 problems are a little tricky to define here. They aren’t necessarily more difficult, they don’t need a context, nor do they need to have more steps.  A Type 2 problem asks students to get to relationships about the concepts involved.  Essentially, Type 2 problems are about asking something where students could have plenty of possible answers (open ended). Again, here is Marian Small describing some examples:

Examples of Type 1 & 2 Questions

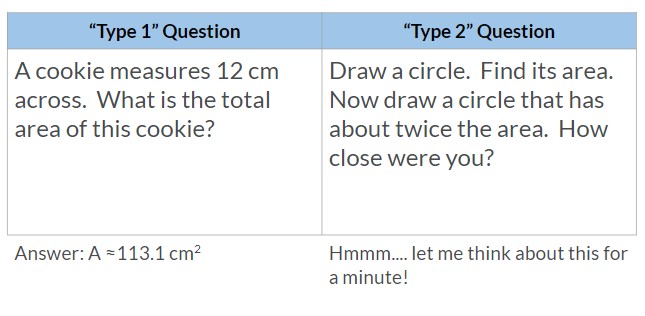
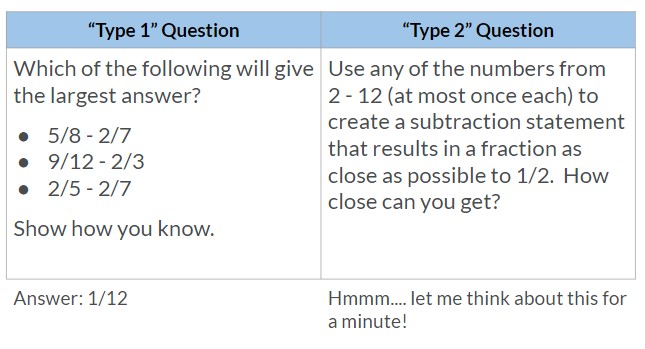
Notice that a type 2 problem is more than just open, it encourages you to keep thinking and try other possibilities!  The constraints are part of what makes this a “type 2” problem! The creativity and interest comes from trying to reach your goal!

Where do you look for “Type 2” Problems?

If you haven’t seen it before, the website called OpenMiddle.com is a great source of Type 2 problems.  Each involve students being creative to solve a potential problem AND start to notice mathematical relationships. 

Home

Remember, mathematically interesting problems (Type 2 problems) are interesting because of the mathematical connections, the relationships involved, the deepening of learning that occurs, not just a fancy context.

Questions to Reflect on:

• When do you include creativity in your math class? All the time? Daily? Toward the beginning of a unit? The end? What does this say about your program? (See A Few Simple Beliefs)
• If you find it difficult to create these types of questions, where do you look? Marian Small is a great start, but there are many places!
• How might “Type 2” problems like these offer your students practice for the skills they have been learning? (See purposeful practice)
• What is the current balance of q]Type 1 and Type 2 problems in your class? Are your students spending more time calculating, or deciding on which calculations are important? What balance would you like?
• How might problems like these help you meet the varied needs within a mixed ability classroom?
• If students start to understand how to solve type 2 problems, would you consider asking your students to make up their own problems? (Ideas for making your own problems here).
• How do these problems help your students build their mathematical intuitions? (See ideas here)
• Would you want students to work alone, in pairs, in groups? Why?
• If you have struggled with developing rich discussions in your class, how might these types of problems help you bring a need for discussions? How might this change class conversations afterward?
• How will you consolidate the learning afterward? (See Never Skip the Closing of the Lesson)
• As the teacher, what will you be doing when students are being creative? How might listening to student thinking help you learn more about your students? (See: Noticing and Wondering: A powerful tool for assessment)

I’d love to continue the conversation about creativity in mathematics.  Leave a comment here or on Twitter @MarkChubb3

Central Tendencies Puzzles

Data management is becoming an increasingly important topic as our students try to make sense of news, social media posts, advertisements… Especially as more and more of these sources aim to try to convince you to believe something (intentionally or not).

Part of our job as math teachers needs to include helping our students THINK as they are collecting / organizing / analyzing data. For example, when looking at data we want our students to:

• Notice the writer’s choice of scale(s)
• Notice the decisions made for categories
• Notice which data is NOT included
• Notice the shape of the data and spatial / proportional connections (twice as much/many)
• Notice the choice of type of graph chosen
• Notice irregularities in the data
• Notice similarities among or between data
• Consider ways to describe the data as a whole (i.e., central tendency) or the story it is telling over time (i.e., trends)

While each of these points are important, I’d like to offer a way we can help our students explore the last piece from above – central tendencies.

Central Tendency Puzzle Templates

To complete each puzzle, you will need to make decisions about where to start, which numbers are most likely and then adjust based on what makes sense or not. I’d love to have some feedback on the puzzles.

Linked here are the Central Tendencies Puzzles.

Questions to Reflect on:

• How will your students be learning about central tendencies before doing these puzzles? What kinds of experience might lead up to these puzzles? (See A Few Simple Beliefs)
• How might puzzles like these offer your students practice for the skills they have been learning? (See purposeful practice)
• How might puzzles like this relate to playing Skyscraper puzzles?
• What is the current balance of questions / problems in your class? Are your students spending more time calculating, or deciding on which calculations are important? What balance would you like?
• How might these puzzles help you meet the varied needs within a mixed ability classroom?
• If students start to understand how to solve one of these, would you consider asking your students to make up their own puzzles? (Ideas for making your own problems here).
• How do these puzzles help your students build their mathematical intuitions? (See ideas here)
• Would you want students to work alone, in pairs, in groups? Why?
• Would you prefer all of your students doing the same puzzle / game / problem, or have many puzzles / games / problems to choose from? How might this change class conversations afterward?
• How will you consolidate the learning afterward? (See Never Skip the Closing of the Lesson)
• As the teacher, what will you be doing when students are playing? How might listening to student thinking help you learn more about your students? (See: Noticing and Wondering: A powerful tool for assessment)

I’d love to continue the conversation about these puzzles.  Leave a comment here or on Twitter @MarkChubb3

Whose problems? Whose game? Whose puzzle?

TRU Math (Teaching for Robust Understanding) a few years ago shared their thoughts about what makes for a “Powerful Classroom”. Here are their 5 dimensions:

Looking through the dimensions here, it is obvious that some of these dimensions are discussed in detail in professional development sessions and in teacher resources. However, the dimension of Agency, Authority and Identity is often overlooked – maybe because it is much more complicated to discuss. Take a look at what this includes:

This dimension helps us as teachers consider our students’ perspectives. How are they experiencing each day? We should be reflecting on:

• Who has a voice? Who doesn’t?
• How are ideas shared between and among students?
• Who feels like they have contributed? Who doesn’t?
• Who is actively contributing? Who isn’t?

Reflecting on our students’ experiences makes us better teachers! So, I’ve been wondering:

Who created today’s problem / game / puzzle?

For most students, math class follows the same pattern:

This pattern of a lesson leaves many students disinterested, because they are not actively involved in the learning – which might lead to typical comments like, “When will we ever need this?”. This lesson format is TEACHER centered because it centers the teachers’ ideas (the teacher provides the problem, the teacher helps students, the teacher tells you if you are correct). In this example, students’ mathematical identities are not fostered. There is NO agency afforded to students. Authority solely belongs to the teachers. But there are ways to make identity / agency / authority a focus!

STUDENT driven ideas

Today as a quick warm-up, I had students solve a little pentomino puzzle.  After they finished, I asked students to create their own puzzles that others will solve.  Here is one of the student created puzzles:

Here you can see a simple puzzle. The pieces are shown that you must use, and the board is included (with a hole in the middle). Now, as a class, we have a bank of puzzles we can attempt any day (as a warm-up or if work is finished).

You can read about WHY we would do puzzles like this in math class along with some examples (Spatial Reasoning).  

What’s more important here is for us to reflect on how we are involving our own students in the creation of problems, games and puzzles in our class.  This is a low-risk way to allow everyone in class do more than just participate, they are taking ownership in their learning, and building a community of learners that value learning WITH and FROM each other!

How to involve our students?

The example above shows us a simple way to engage our students, to expand what we consider mathematics and help our students form positive mathematical identities. However, there are lots of ways to do this:

• Play a math game for a day or 2, then ask students to alter one or a few of the rules.
• Have students submit questions you might want to consider for an assessment opportunity.
• Have students look through a bank or questions / problems and ask which one(s) would be the most important ones to do.
• Give students a sheet of many questions. Ask them to only do the 3 easiest, and the 3 hardest (then lead a discussion about what makes those ones the hardest).
• Lead 3-part math lessons where students start by noticing / wondering.
• Have students design their own SolveMe mobile puzzles, visual patterns, Which One Doesn’t Belong…

Questions to Reflect on:

• Who is not contributing in your class, or doesn’t feel like they are a “math student”? Whose mathematical identities would you like to foster? How might something simple like this make a world of difference for those children?
• Does it make a difference WHO develops the thinking?
• Fostering student identities, paying attention to who has authority in your class and allowing students to take ownership is essential to build mathematicians. The feeling of belonging in this space is crucial. How are you paying attention to this? (See Matthew Effect)
• How might these ideas help you meet the varied needs within a mixed ability classroom?
• If you do have your students create their own puzzles, will you first offer a simplified version so your students get familiar with the pieces, or will you dive into having them make their own first?
• Would you prefer all of your students doing the same puzzle / game / problem, or have many puzzles / games / problems to choose from? How might this change class conversations afterward?
• As the teacher, what will you be doing when students are playing? How might listening to student thinking help you learn more about your students? (See: Noticing and Wondering: A powerful tool for assessment)

I’d love to continue the conversation about students’ identities in mathematics.  Leave a comment here or on Twitter @MarkChubb3

“Number Boxes”

A few weeks ago I was introduced to Jenna Laib‘s game “Number Boxes” and was very interested in using it as a dynamic game to help students learn a variety of new content — Jenna’s blog explaining the game can be found here: “One of My Favorite Games: Number Boxes“.

Basically the game involves students rolling dice (or spinning a spinner / drawing a card) to generate a random number and placing that number in one of their empty number boxes one-at-a-time. The game can progress in a variety of ways:

Rolling 1 number at a time, create the largest number you can.

Rolling one number at a time, create 2 numbers that will add to the largest number.

Rolling one number at a time, create an expression that is as close to 2000 as possible.

As you can see, the game is quite adaptive to the sizes of numbers and concepts your students are comfortable with. As students roll/spin/draw a number, they have to place it on the board. What makes this tricky is not knowing what future numbers will be. In the board above, you can see that there is also a “Throwaway” box that students can use if they do not like one of the numbers rolled/spun/drawn. This game is an excellent example of a “Dynamic Game” or “Dynamic Practice” as students are following the ideals on the right side of the chart below:

Originally published here

Blow is a gallery of some possible adaptations of this game or linked here is a slideshow

• 

• 

• 

• 

• 

• 

• 

• 

Metric Conversions

I however, wanted to use Jenna’s game to help students practice a concept they often have difficulty with – Metric Conversions. Once students have had many opportunities to estimate and measure various distances, capacities, and masses, they should be able to start making connections between all of the units. I suggest a good balance between using problems that help students make sense of the relationships between the units, and opportunities to practice conversions on their own. However, instead of randomly generated worksheets or other rote practice, I think Jenna’s game could work perfectly. Take a look at some examples:

Rolling one number at a time, find the largest total distance possible

Rolling one number at a time, find the largest total mass possible

Rolling one number at a time, find the largest possible distance

Rolling one number at a time, how close to 5km can you get?

Reflection

It is important to offer tasks that allow students to make choices and decisions like the ones offered in this game. Learning needs to be more than handing out assignments, and collecting work… Learning takes time! Students need more time to explore, see what works, have peers challenge each others’ thinking, make important connections… Hopefully you can see these opportunities in this task.

Final Thoughts:

• If you play one of these games, or your own version, will you first offer a simplified version so your students get familiar with the game, or will you dive into the content you want to teach?
• Would you prefer your students to play this game as a class or with a group, a partner, or independently?
• How will you build in conversations with students so they discuss which numbers they think should be the highest / lowest numbers? How will you offer time for these strategic discussions?
• Should we adapt these to continually offer more challenge and deeper learning, or offer more opportunities to play the same game board? How will we know when to adapt and change?
• What does “practice” look like in your classroom?  Does it involve thinking or decisions?  Would it be more engaging for your students to make practice involve more thinking?
• How does this game relate to the topic of “engagement”?  Is engagement about making tasks more fun or about making tasks require more thought?  Which view of engagement do you and your students subscribe to?
• How have your students experienced measurement concepts like these? Are they learning procedural rules or are they thinking about the actual sizes of numbers / sizes of the units involved?
• As the teacher, what will you be doing when students are playing? How might listening to student thinking help you learn more about your students? (See: Noticing and Wondering: A powerful tool for assessment)

I’d love to continue the conversation about “practicing” mathematics.  Leave a comment here or on Twitter @MarkChubb3

An Example of Teaching THROUGH problem solving?

Many math resources attempt to share the difference between teaching FOR problem solving and teaching THROUGH problem solving. Cathy Seeley refers to teaching THROUGH problem solving as “Upside-Down Teaching” which is the opposite of a “gradual release of responsibility” model:

And instead calls for us to flip how our students learn to a more active model:

So, instead of starting a unit on Geometry with naming shapes or developing definitions together, we decided to start with a little problem:

Create as many polygons as possible using exactly 2 pattern block pieces. Sort your polygons by how many sides they have.

As students started placing pattern block pieces together, all kinds of questions started emerging (questions we took note of to bring to the whole group in a few minutes):

What is this shape called? It has 6 sides, so is it a hexagon?

This shape has 12 sides. Am I allowed to do this?

Are these the same shapes or different? Do I have to line up the sides or can I place a shape in the middle like I did here (on the right)?

By the end of a period, students had worked through the definitions of what a polygon is (and isn’t), the difference between concave and convex polygons, defined the term “regular polygon” (which was not what they had been calling “regular” before), and were able to name and create triangles, quadrilaterals, pentagons, hexagons, heptagons, octagons, nonagons, decagons and undecagons. Recognizing a variety of possible ways a shape can look was very helpful for our students who might have experienced shapes more traditionally in the past.

One group compiled their polygons together (with one minor error):

Instead of starting with experiences where students accumulate knowledge (writing out definitions, taking a note, direct instruction), an upside down approach aims to start with students’ ideas. This way we would know which conversations to have with our students, and so our students are actively engaged in the process of learning.

I want to leave you with a few reflective questions:

• Why might it benefit students to start with a problem instead of starting with the teachers’ ideas?
• Why might it benefit teachers to listen to students’ thinking before instruction has occurred?
• What does it mean to effectively monitor students as they are thinking / working? (See This POST for examples)
• Can all mathematics topics begin with tasks that help our students make connections between what they already know, and what they are learning? Can you think of a topic that can not be experienced this way?
• The final stage in the You-We-I model is where the teacher helps make specific learning explicit for their students. How do you find time to consolidate a task like this? How do you know what to share? (See This POST for an example)
• How might this form of teaching relate to how we view assessment? (See This POST)
• How might this form of teaching relate to how we view differentiated instruction? (See This POST)
• How do you find problems that ask students to actively think before any instruction has occurred? (See This POST for examples)

I’d love to continue the conversation.  Feel free to write a response, or send me a message on Twitter ( @markchubb3 ).

Spatial Puzzles: Cuisenaire Cover-ups

Foundational to almost every aspect of mathematics is the idea that things can be broken down into pieces or units in a variety of ways, and then be recomposed again. For example, the number 10 can be thought of as 2 groups of 5, or 5 groups of 2, or a 7 and a 3, or two-and-one-half and seven-and-one-half…

Earlier this year I shared a post discussing how we might decompose and recompose numbers to do an operations (subtraction). But, I would like us to consider why some students are more comfortable decomposing and recomposing, and how we might be aiming to help our students early with experiences that might promote the kinds of thinking needed.

Doug Clements and Julie Sarama have looked at the relationship between students’ work with space and shapes with students understanding of numbers.

“The ability to describe, use, and visualize the effects of putting together and taking apart shapes is important because the creating, composing, and decomposing units and higher-order units are fundamental mathematics. Further, there is transfer: Composition of shapes supports children’s ability to compose and decompose numbers”

Contemporary Perspectives on Mathematics in Early Childhood Education p.82, Clements and Sarama

The connection between composing and decomposing shapes and numbers is quite exciting to me. However, I am also very interested in the meeting place between Spatial tasks (composing/decomposing shapes) and Number tasks that involve composing and decomposing.

A few years ago I found a neat little puzzle in a resource called The Super Source called “Cover the Giraffe”. The idea was to cover an image of a giraffe outline using exactly 1 of each size of cuisenaire rods. The task, simple enough, was actually quite difficult for students (and even for us as adults). After using the puzzle with a few different classes, I decided to make a few of my own.

After watching a few classrooms of students complete these puzzles, I noticed an interesting intersection between spatial reasoning, and algebraic reasoning happening…. First, let me share the puzzles with you:

Objective:

To complete a Cuisenaire Cover-Up puzzle, you need exactly 1 of each colour cuisenaire rod. Use each colour rod once each to completely fill in the image.

Below are the 5 puzzles:

• Cover the Dog
• Cover the Elephant
• Cover the Giraffe (edited from the SuperSource)
• Cover the Rocket
• Cover the Sailboat

Assessment Opportunities

Knowing what to look for, helps us know how to interact with our students.

• Which block are students placing first? The largest blocks or the smallest?
• Which students are using spatial cues (placing rods to see which fits) and which students are using numerical cues (counting units on the grid)? How might we help students who are only using one of these cueing systems without over-scaffolding or showing how WE would complete the puzzle?
• How do our students react when confronted with a challenging puzzle?
• Who is able to swap out 1 rod for 2 rods of equivalent length (1 orange rod is the same length as a brown and red rod together)?
• Which of the following strands of proficiency might you be noting as you observe students:

Adding It Up, 2001

Questions to Reflect on:

• Why might you use a task like this? What would be your goal?
• How will you interact with students who struggle to get started, or struggle to move passed a specific hurdle?
• How might these puzzles relate to algebraic reasoning? (try to complete one with this question in mind)
• How are you making the connections between spatial reasoning and algebraic reasoning clear for your students to see? How can these puzzles help?
• How might puzzles allow different students to be successful in your class?

I’d love to continue the conversation about how we can use these puzzles to further our students’ spatial/algebraic reasoning.  Leave a comment here or on Twitter @MarkChubb3

If interested in these puzzles, you might be interested in trying:

• Zukei Puzzles
• Skyscraper Templates for Relational Rods
• Creating Voronoi
• Skyscraper Templates
• Making Math Visual

“The More Strategies, the Better?

As many teachers implement number talks/math strings and lessons where students are learning through problem solving, the idea that there are many ways to answer a question or problem becomes more important. However, I think we need to unpack the beliefs and practices surrounding what it means for our students to have different “strategies”. A few common beliefs and practices include:

Really, there are benefits and issues with each of these thoughts…. and the right answer is actually really much more complicated than any of these.  To help us consider where our own decisions lie, let’s start by considering an actual example. If students were given a pattern with the first 4 terms like this:

See VisualPatterns.org for more visual patterns

…and asked how many shapes there would be on the 24th design (how many squares and circles in total).  Students could tackle this in many ways:

• Draw out the 24th step by building on and keeping track of each step number
• Build the 24th step by adding on and keeping track of the step number
• Make a T-table and use skip counting to find each new step (5, 9, 13, 17…).
• Find the explicit rule from the first few images’ data placed on a T-table (“I see the pattern is 5, 9, 13, 17. each new image uses 4 new shapes, so the pattern is a multiplied by 4 pattern…. and I think the rule should be ‘number of images = step number x4+1’. Let me double check…”).
• Notice the “constant” and multiplicative aspects of the visual, then find the explicit rule (I see that each image increases by 4 new shapes on the right, so the multiplicative aspect of this pattern is x4, and term 0 might just be 1 circle. So the pattern must be x4+1″).
• Create a graph, then find the explicit rule based on starting point and growth (“When I graph this, my line hits the y-axis at 1, and increases by 4 each time, so the pattern rule must be x4+1”).

While each of these might offer a correct answer, we as the teacher need to assess (figure out what our students are doing/thinking) and then decide on how to react accordingly.  If a student is using an additive strategy (building each step, or creating a t-table with every line recorded using skip counting), their strategy is a very early model of understanding here and we might want to challenge this/these students to find or use other methods that use multiplicative reasoning.  Saying “do it another way” might be helpful here, but it might not be helpful for other students.  If on the other hand, a student DID use multiplicative reasoning, and we suggest “do it another way”, then they fill out a t-table with every line indicated, we might actually be promoting the use of less sophisticated reasoning.  

On the other hand, if we tell/show students exactly how to find the multiplicative rule, and everyone is doing it well, then I would worry that students would struggle with future learning.  For example, if everyone is told to make a t-table, and find the recursive pattern (above would be a recursive pattern of+4 for total shapes), then use that as the multiplicative basis for the explicit rule x4 to make x4+1), then students are likely just following steps, and are not internalizing what specifically in the visual pattern here is +4 or x4… or where the constant of +1 is.  I would expect these students to really struggle with figuring out patterns like the following that is non-linear:

See VisualPatterns.org for more visual patterns

Students told to start with a t-table and find the explicit pattern rule are likely not even paying attention to what in the visual is growing, how it is growing or what is constant between each figure. So, potentially, moving students too quickly to the most sophisticated models will likely miss out on the development necessary for them to be successful later.

While multiple strategies are helpful to know, it is important for US to know which strategies are early understandings, and which are more sophisticated.  WE need to know which students to push and when to allow everyone to do it THEIR way, then hold a math congress together to discuss relationships between strategies, and which strategies might be more beneficial in which circumstance. It is the relationships between strategies that is the MOST important thing for us to consider!

Focusing on OUR Understanding:

In order for us to know which sequence of learning is best for our students, and be able to respond to our students’ current understandings, we need to be aware of how any particular math concepts develops over time. Let’s be clear, understanding and using a progression like this takes time and experience for US to understand and become comfortable with.

While most educational resources are filled with lessons and assessment opportunities, very few offer ideas for us as teachers about what to look for as students are working, and how to respond to different students based on their current thinking. This is what Deborah Ball calls “Math Knowledge for Teaching”:

If any teacher wants to improve their practice, I believe this is the space that will have the most impact! If schools are interested in improving math instruction, helping teachers know what to look for, and how to respond is likely the best place to tackle. If districts are aiming for ways to improve, helping each teacher learn more about these progressions will likely be what’s going to make the biggest impacts!

Where to Start?

If you want to deepen our understanding of the math we teach, including better understanding how math develops over time, I would suggest:

• Providing more open questions, and looking at student samples as a team of teachers
• Using math resources that have been specifically designed with progressions in mind (Cathy Fosnot’s Contexts for Learning and minilessons, Cathy Bruce & Ruth Beatty’s From Patterns to Algebra, Alex Lawson’s What to Look For…), and monitoring student strategies over time
• Anticipating possible student strategies, and using a continuum or landscape (Cathy Fosnot’s Landscapes, Lawson’s Continua, Clement’s Trajectories, Van Hiele’s levels of geometric thought…) as a guide to help you see how your students are progressing
• Collaborate with other educators using resources designed for teachers to deepen their understanding and provide examples for us to use with kids (Marian Small’s Understanding the Math we Teach, Van de Walle’s Teaching Student Centered Mathematics, Alex Lawsons’s What to Look For, Doug Clements’ Learning and Teaching Early Math…)
• Have discussions with other math educators about the math you teach and how students develop over time.

Questions to Reflect on:

• How do you typically respond to your students when you give them opportunities to share their thinking? Which of the 3 beliefs/practices is most common for you? How might this post help you consider other beliefs/practices?
• How can you both honour students’ current understandings, yet still help students progress toward more sophisticated understandings?
• Given that your students’ understandings at the beginning of any new learning differ greatly, how do you both learn about your students’ thoughts and respond to them in ways that are productive? (This is different than testing kids prior knowledge or sorting students by ability. See Daro’s video)
• Who do you turn to to help you think more about the math you teach, or they ways you respond to students? What professional relationships might be helpful for you?
• What resources do you consult to help you develop your own understanding?

I’d love to continue the conversation about how we respond to our students’ thinking.  Leave a comment here or on Twitter @MarkChubb3

If interested in this topic, you might be interested in reading:

• Strategies vs Models
• Professional Development: what should it look like?
• Paying Attention to OUR Understanding
• Noticing and Wondering: a powerful tool for assessment

Decomposing & Recomposing – How we subtract

Throughout mathematics, the idea that objects and numbers can be decomposed and recomposed can be found almost everywhere. I plan on writing a few articles in the next while to discuss a few of these areas. In this post, I’d like to help us think about how and why we use visual representations and contexts to help our students make sense of the numbers they are using.

Decomposing and Recomposing

Foundational to almost every aspect of mathematics is the idea that things can be broken down into pieces or units in a variety of ways, and be then recomposed again. For example, the number 10 can be thought of as 2 groups of 5, or 5 groups of 2, or a 7 and a 3, or two-and-one-half and seven-and-one-half…

Understanding how numbers are decomposed and recomposed can help us make sense of subtraction when we consider 52-19 as being 52-10-9 or 52-20+1 or (40-10)+(12-9) or 49-19+3 (or many other possibilities)… Let’s take a look at how each of these might be used:

The traditional algorithm suggests that we decompose 52-19 based on the value of each column, making sure that each column can be subtracted 1 digit at a time… In this case, the question would be recomposed into (40-10)+(12-9). Take a look:

52 is decomposed into 40+10+2
19 is decomposed into 10+9
The problem is recomposed into (40-10) + (12-9)

While this above strategy makes sense when calculating via paper-and-pencil, it might not be helpful for our students to develop number sense, or in this case, maintain magnitude. That is, students might be getting the correct answer, but completely unaware that they have actually decomposed and recomposed the numbers they are using at all.

Other strategies for decomposing and recomposing the same question could look like:

Maintain 52
Decompose 19 into 10+9
Subtract 52-10 (landing on 42), then 42-9
Some students will further decompose 9 as 2+7 and recompose the problem as 42-2-7

Maintain 52
Decompose 19 as 20-1
Recompose the problem as 52-20+1

Decompose 52 as 49-3
Recompose the problem as 49-19+3

The first problem at the beginning was aimed at helping students see how to “regroup” or decompose/recompose via a standardized method. However, the second and third examples were far more likely used strategies for students/adults to use if using mental math. The last example pictured above, illustrates the notion of “constant difference” which is a key strategy to help students see subtraction as more than just removal (but as the difference). Constant difference could have been thought of as 52-19 = 53-20 or as 52-19 = 50-17, a similar problem that maintains the same difference between the larger and smaller values. Others still, could have shown a counting-on strategy (not shown above) to represent the relationship between addition and subtraction (19+____=53).

Why “Decompose” and “Recompose”?

The language we use along with the representations we want from our students matters a lot. Using terms like “borrowing” for subtraction does not share what is actually happening (we aren’t lending things expecting to receive something back later), nor does it help students maintain a sense of the numbers being used. Liping Ma’s research, shared in her book Knowing and Teaching Elementary Mathematics, shows a comparison between US and Chinese teachers in how they teach subtraction. Below you can see that the idea of regrouping, or as I am calling decomposing and recomposing, is not the norm in the US.

Visualizing the Math

There seems to be conflicting ideas about how visuals might be helpful for our students. To some, worksheets are handed out where students are expected to draw out base 10 blocks or number lines the way their teacher has required. To others, number talks are used to discuss strategies kids have used to answer the same question, with steps written out by their teachers.

In both of these situations, visuals might not be used effectively. For teachers who are expecting every student to follow a set of procedures to visually represent each question, I think they might be missing an important reason behind using visuals. Visuals are meant to help our students see others’ ideas to learn new strategies! The visuals help us see What is being discussed, Why it works, and How to use the strategy in the future.

Teachers who might be sharing number talks without visuals might also be missing this point. The number talk below is a great example of explaining each of the types of strategies, but it is missing a visual component that would help others see how the numbers are actually being decomposed and recomposed spatially.

If we were to think developmentally for a moment (see Dr. Alex Lawson’s continuum below), we should notice that the specific strategies we are aiming for, might actually be promoted with specific visuals. Those in the “Working with the Numbers” phase, should be spending more time with visuals that help us SEE the strategies listed.

Aiming for Fluency

While we all want our students to be fluent when using mathematics, I think it might be helpful to look specifically at what the term “procedural fluency” means here. Below is NCTM’s definition of “procedural fluency” (verbs highlighted by Tracy Zager):

Which of the above verbs might relate to our students being able to “decompose” and “recompose”?

Some things to think about:

• How well do your students understand how numbers can be decomposed and recomposed? Can they see that 134 can be thought of as 1 group of 100, 3 groups of 10, and 4 ones AS WELL AS 13 groups of 10, and 4 ones, OR 1 group of 100, 2 groups of 10, and 14 ones…….? To decompose and recompose requires more than an understanding of digit values!!!
• How do the contexts you choose and the visual representations you and your students use help your students make connections? Are they calculating subtraction questions, or are they thinking about which strategy is best based on the numbers given?
• What developmental continuum do you use to help you know what to listen for?
• How much time do your students spend calculating by hand? Mentally figuring out an answer? Using technology (a calculator)? What is your balance?
• How might the ideas of decomposing and recomposing relate to other topics your students have learned and will learn in the future?
• Are you teaching your students how to get an answer, or how to think?

If you are interested in learning more, I would recommend:

• The Importance of Contexts and Visuals
• How to make math visual
• The difference between Strategies and Models

I’d love to continue the conversation about assessment in mathematics.  Leave a comment here or on Twitter @MarkChubb3

Rushing for Interventions

I see students working in groups all the time…  Students working collaboratively in pairs or small groups having rich discussions as they sort shapes by specific properties, students identifying and extending their partner’s visual patterns, students playing games aimed at improving their procedural fluency, students cooperating to make sense of a low-floor/high-ceiling problem…..

When we see students actively engaged in rich mathematics activities, working collaboratively, it provides opportunities for teachers to effectively monitor student learning (notice students’ thinking, provide opportunities for rich questioning, and lead to important feedback and next steps…) and prepare the teacher for the lesson close.  Classrooms that engage in these types of cooperative learning opportunities see students actively engaged in their learning.  And more specifically, we see students who show Agency, Ownership and Identity in their mathematics learning (See TruMath‘s description on page 10).

---

On the other hand, some classrooms might be pushing for a different vision of what groups can look like in a mathematics classroom.  One where a teachers’ role is to continually diagnose students’ weaknesses, then place students into ability groups based on their deficits, then provide specific learning for each of these groups.  To be honest, I understand the concept of small groups that are formed for this purpose, but I think that many teachers might be rushing for these interventions too quickly.

First, let’s understand that small group interventions have come from the RTI (Response to Intervention) model.  Below is a graphic created by Karen Karp shared in Van de Walle’s Teaching Student Centered Mathematics to help explain RTI:

Response to Intervention – Teaching Student Centered Mathematics

As you can see, given a high quality mathematics program, 80-90% of students can learn successfully given the same learning experiences as everyone.  However, 5-10% of students (which likely are not always the same students) might struggle with a given topic and might need additional small-group interventions.  And an additional 1-5% might need might need even more specialized interventions at the individual level.

The RTI model assumes that we, as a group, have had several different learning experiences over several days before Tier 2 (or Tier 3) approaches are used.  This sounds much healthier than a model of instruction where students are tested on day one, and placed into fix-up groups based on their deficits, or a classroom where students are placed into homogeneous groupings that persist for extended periods of time.

---

Principles to Action (NCTM) suggests that what I’m talking about here is actually an equity issue!

Principles to Action

We know that students who are placed into ability groups for extended periods of time come to have their mathematical identity fixed because of how they were placed.  That is, in an attempt to help our students learn, we might be damaging their self perceptions, and therefore, their long-term educational outcomes.

---

Tier 1 Instruction

While I completely agree that we need to be giving attention to students who might be struggling with mathematics, I believe the first thing we need to consider is what Tier 1 instruction looks like that is aimed at making learning accessible to everyone.  Tier 1 instruction can’t simply be direct instruction lessons and whole group learning.  To make learning mathematics more accessible to a wider range of students, we need to include more low-floor/high-ceiling tasks, continue to help our students spatalize the concepts they are learning, as well as have a better understanding of developmental progressions so we are able to effectively monitor student learning so we can both know the experiences our students will need to be successful and how we should be responding to their thinking.  Let’s not underestimate how many of our students suffer from an “experience gap”, not an “achievement gap”!

If you are interested in learning more about what Tier 1 instruction can look like as a way to support a wider range of students, please take a look at one of the following:

•  How do we meet the needs of so many unique students in a mixed-ability classroom?
• Differentiated Instruction: comparing 2 subjects
• Differentiating Mathematics Instruction

---

Tier 2 Instruction

Tier 2 instruction is important.  It allows us to give additional opportunities for students to learn the things they have been learning over the past few days/weeks in a small group.  Learning in a small group with students who are currently struggling with the content they are learning can give us opportunities to better know our students’ thinking.  However, I believe some might be jumping past Tier 1 instruction (in part or completely) in an attempt to make sure that we are intervening. To be honest, this doesn’t make instructional sense to me! If we care about our content, and care about our students’ relationship with mathematics, this might be the wrong first move.

So, let’s make sure that Tier 2 instruction is:

• Provided after several learning experiences for our students
• Flexibly created, and easily changed based on the content being learned at the time
• Focused on student strengths and areas of need, not just weaknesses
• Aimed at honoring students’ agency, ownership and identity as mathematicians
• Temporary!

If you are interested in learning more about what Tier 2 interventions can look like take a look at one of the following:

• RTI for Adults
• Targeted Instruction
• Continuing to get better together

---

Instead of seeing mathematics as being learned every day as an approach to intervene, let’s continue to learn more about what Tier 1 instruction can look like!  Or maybe you need to hear it from John Hattie:

Or from Jo Boaler:

---

Final Thoughts

If you are currently in a school that uses small group instruction in mathematics, I would suggest that you reflect on a few things:

• How do your students see themselves as mathematicians?  How might the topics of Agency, Authority and Identity relate to small group instruction?
• What fixed mindset messaging do teachers in your building share “high kids”, “level 2 students”, “she’s one of my low students”….?  What fixed mindset messages might your students be hearing?
• When in a learning cycle do you employ small groups?  Every day?  After several days of learning a concept?
• How flexible are your groups?  Are they based on a wholistic leveling of your students, or based specifically on the concept they are learning this week?
• How much time do these small groups receive?  Is it beyond regular instructional timelines, or do these groups form your Tier 1 instructional time?
• If Karp/Van de Walle suggests that 80-90% of students can be successful in Tier 1, how does this match what you are seeing?  Is there a need to learn more about how Tier 1 approaches can meet the needs of this many students?
• What are the rest of your students doing when you are working with a small group?  Is it as mathematically rich as the few you’re working with in front of you?
• Do you believe that all of your students are capable to learn mathematics and to think mathematically?

I’d love to continue the conversation.  Write a response, or send me a message on Twitter ( @markchubb3 ).

Noticing and Wondering: A powerful tool for assessment

Last week I had the privilege of presenting with Nehlan Binfield at OAME on the topic of assessment in mathematics.  We aimed to position assessment as both a crucial aspect of teaching, yet simplify what it means for us to assess effectively and how we might use our assessments to help our students and class learn.  If interested, here is an abreviated version of our presentation:

We started off by running through a Notice and Wonder with the group.  Given the image above, we noticed colours, sizes, patterns, symmetries (line symmetry and rotational symmetry), some pieces that looked like “trees” and other pieces that looked like “trees without stumps”…

Followed by us wondering about how many this image would be worth if a white was equal to 1, and what the next term in a pattern would look like if this was part of a growing pattern…

We didn’t have time, but if you are interested you can see the whole exchange of how the images were originally created in Daniel Finkel’s quick video.

We then continued down the path of noticing and wondering about the image above.  After several minutes, we had come together to really understand the strategy called Notice and Wonder:

As well as taking a quick look at how we can record our students’ thinking:

Shared by Jamie Duncan

---

At this point in our session, we changed our focus from Noticing and Wondering about images of mathematics, to noticing and wondering about our students’ thinking.  To do this, we viewed the following video (click here to view) of a student attempting to find the answer of what eight, nine-cent stamps would be worth:

The group noticed the student in the video counting, pausing before each new decade, using two hands to “track” her thinking…  The group noticed that she used most of a 10-frame to think about counting by ones into groups of 9.

We then asked the group to consider the wonders about this student or her thinking and use these wonders to think about what they would say or do next.

• Would you show her a strategy?
• Would you ask a question to help you understand their thinking better?
• Would you suggest a tool?
  Would you give her a different question?

It seemed to us, that the most common next steps might not be the ones that were effectively using our assessment of what this child was actually doing.

Looking through Fosnot’s landscape we noticed that this student was using a “counting by ones” strategy (at least when confronted with 9s), and that skip-counting and repeated addition were the next strategies on her horizon.

While many teachers might want to jump into helping and showing, we invited teachers to first consider whether or not we were paying attention to what she WAS actually doing, as opposed to what she wasn’t doing.

---

This led nicely into a conversation about the difference between Assessment and Evaluation.  We noticed that we many talk to us about “assessment”, they actually are thinking about “evaluation”.  Yet, if we are to better understand teaching and learning of mathematics, assessment seems like a far better option!

So, if we want to get better at listening interpretively, then we need to be noticing more:

Yet still… it is far too common for schools to use evaluative comments.  The phrases below do not sit right with me… and together we need to find ways to change the current narrative in our schools!!!

Evaluation practices, ranking kids, benchmarking tests… all seem to be aimed at perpetuating the narrative that some kids can’t do math… and distracts us from understanding our students’ current thinking.

So, we aimed our presentation at seeing other possibilities:

---

To continue the presentation, we shared a few other videos of student in the processs of thinking (click here to view the video).  We paused the video directly after this student said “30ish” and asked the group again to notice and wonder… followed by thinking about what we would say/do next. 

Followed by another quick video (click here to view).  We watched the video up until she says “so it’s like 14…”.  Again, we noticed and wondered about this students’ thinking… and asked the group what they would say or do next.

After watching the whole video, we discussed the kinds of questions we ask students:

If we are truly aimed at “assessment”, which basically is the process of understanding our students’ thinking, then we need to be aware of the kinds of questions we ask, and our purpose for asking those questions!  (For more about this see link).

---

We finished our presentation off with a framework that is helpful for us to use when thinking about how our assessment data can move our class forward:

We shared a selection of student work and asked the group to think about what they noticed… what they wonderered… then what they would do next.

For more about how the 5 Practices can be helpful to drive your instruction, see here.

---

So, let’s remember what is really meant by “assessing” our students…

…and be aware that this might be challenging for us…

…but in the end, if we continue to listen to our students’ thinking, ask questions that will help us understand their thoughts, continue to press our students’ thinking, and bring the learning together in ways where our students are learning WITH and FROM each other, then we will be taking “a giant step toward becoming a master teacher”!

---

So I’ll leave you with some final thoughts:

• What do comments sound like in your school(s)?  Are they asset based (examples of what your students ARE doing) or deficit based (“they can’t multiply”… “my low kids don’t get it…”)?
• What do you do if you are interested in getting better at improving your assessment practices like we’ve discussed here, but your district is asking for data on spreadsheets that are designed to rank kids evaluatively?
• What do we need to do to change the conversation from “level 2 kids” (evaluative statements that negatively impact our students) to conversations about what our students CAN do and ARE currently doing?
•  What math knowledge is needed for us to be able to notice mathematicially important milestones in our students?  Can trajectories or landscapes or continua help us know what to notice better?

---

I’d love to continue the conversation about assessment in mathematics.  Leave a comment here or on Twitter @MarkChubb3 @MrBinfield

---

If you are interested in reading more on similar topics, might I suggest:

• Questioning the pattern of our questions
• Starting where our students are….. with THEIR thoughts
• How do we meet the needs of so many unique students in a mixed-ability classroom?
• Never Skip the Closing of the Lesson
• What does “Assessment Drives Learning” mean to you?
• Who makes the biggest impact?

Or take a look at the whole slide show here

 

The Importance of Contexts and Visuals

My wife Anne-Marie isn’t always impressed when I talk about mathematics, especially when I ask her to try something out for me, but on occasion I can get her to really think mathematically without her realizing how much math she is actually doing.  Here’s a quick story about one of those times, along with some considerations:

---

A while back Anne-Marie and I were preparing lunch for our three children.  It was a cold wintery day, so they asked for Lipton Chicken Noodle Soup.  If you’ve ever made Lipton Soup before you would know that you add a package of soup mix into 4 cups of water.

Typically, my wife would grab the largest of our nesting measuring cups (the one marked 1 cup), filling it four times to get the total required 4 cups, however, on this particular day, the largest cup available was the 3/4 cup.

Here is how the conversation went:

Anne-Marie:  How many of these (3/4 cups) do I need to make 4 cups?

Me:  I don’t know.  How many do you think?  (attempting to give her time to think)

Anne-Marie:  Well… I know two would make a cup and a half… so… 4 of these would make 3 cups…

Me: OK…

Anne-Marie:  So, 5 would make 3 and 3/4 cups.

Me:  Mmhmm….

Anne-Marie:  So, I’d need a quarter cup more?

Me:  So, how much of that should you fill?  (pointing to the 3/4 cup in her hand)

Anne-Marie:  A quarter of it?  No, wait… I want a quarter of a cup, not a quarter of this…

Me:  Ok…

Anne-Marie:  Should I fill it 1/3 of the way?

Me:  Why do you think 1/3?

Anne-Marie:  Because this is 3/4s, and I only need 1 of the quarters.

---

The example I shared above illustrates sense making of a difficult concept – division of fractions – a topic that to many is far from our ability of sense making.  My wife, however, quite easily made sense of the situation using her reasoning instead of a formula or an algorithm.  To many students, however, division of fractions is learned first through a set of procedures.

I have wondered for quite some time why so many classrooms start with procedures and algorithms unill I came across Liping Ma’s book Knowing and Teaching Mathematics.  In her book she shares what happened when she asked American and Chinese teachers these 2 problems:

Here were the results:

Now, keep in mind that the sample sizes for each group were relatively small (23 US teachers and 72 Chinese teachers were asked to complete two tasks), however, it does bring bring about a number of important questions:

• How does the training of American and Chinese teachers differ?
• Did both groups of teachers rely on the learning they had received as students, or learning they had received as teachers?
• What does it mean to “Understand” division of fractions?  Computing correctly?  Beging able to visually represent what is gonig on when fractions are divided? Being able to know when we are being asked to divide?  Being able to create our own division of fraction problems?
• What experiences do we need as teachers to understand this concept?  What experiences should we be providing our students?

---

Visual Representations

In order to understand division of fractions, I believe we need to understand what is actually going on.  To do this, visuals are a necessity!  A few examples of visual representations could include:

A number line:

A volume model:

An area model:

---

Starting with a Context

Starting with a context is about allowing our students to access a concept using what they already know (it is not about trying to make the math practical or show students when a concept might be used someday).  Starting with a context should be about inviting sense-making and thinking into the conversation before any algorithm or set of procedures are introduced.  I’ve already shared an example of a context (preparing soup) that could be used to launch a discussion about division of fractions, but now it’s your turn:

Design your own problem that others could use to launch a discussion of division of fractions.  Share your problem!  

---

A few things to reflect on:

• How do you use contexts and visuals to help your students make sense of concepts?
• What does day 1 look like for any new concept in your classroom?
• How do you know if your students “understand” a topic?  How would you define “understanding“?
• How do you assess your students’ understanding?  How does this assessment help you know where to go next?
• How do we get better as teachers to understand the mathematics we teach?
• If you know that a specific concept is difficult for you to visually represent, where do you turn to continue your own learning?

As always, I’d love to hear your thoughts.  Leave a reply here on Twitter (@MarkChubb3)

How Many Do You See (Part 2 of 2)

A few weeks ago I shared with you a quick blog post showing a simple worksheet at the grade 2 level – the kind of  simple worksheet that is common to many classrooms.  If you haven’t seen the image, here it is again:

As you can see, the task asks students to correctly count the number of each shape they notice.  In my first post (Part 1) I asked us a few questions to start a conversation:

1. Pick one shape (or more if you’re adventurous)
2. Think about what you believe the teacher’s edition would say
3. Count how many you see
4. Share the 3 points above as a comment here or on Twitter

I was quite happy with where some of the conversations led…

Some of the conversations revolved around the issue many have with resources perpetuating stereotypical definitions of shapes:

If we look, there are exactly 4 shapes that resemble the diagram at the top of the page labelled as “rectangle”, however, there are several different sized squares as well (a square are a special case of a rectangle).

---

Other conversations revolved around actually counting the number of each item:

What interests me here is that we, as a group of math teachers, have answered this grade 2 worksheet with various answers.  Which brings about 2 important conversations:

1. What are we looking for when students complete a worksheet or textbook questions?
2. Are we aiming for convergent or divergent thinking?  Which of these is more helpful for our students?

---

What are we looking for?

Given the conversations I have had with math teachers about the worksheet being shared here, it seems like there are a few different beliefs.  Some teachers believe the activity is aimed at helping students recognize traditional shapes and identify them on the page.  Other teachers believe that this activity could potentially lead to discussions about definitions of shapes (i.e., What is a rectangle?  What is a hexagon?…) if we listen to and notice our students’ thinking about each of the shapes, then bring students together to have rich discussions.

It’s probably worth noting that the Teacher’s Edition for this worksheet includes precise answers.  If a typical teacher were to collect the students’ work and begin marking the assignment using the “answers” from the teacher’s guide, some of the students would have the “correct” answer of 8 trapezoids, but many others would likely have noticed several of the other trapezoids on the page.  If we are looking / listening for students to find the correct answer, we are likely missing out on any opportunity to learn about our students, or offer any opportunity for our students to learn themselves!

I would hope that an activity like this would provide us opportunities for our students to show what they understand, and move beyond getting answers into the territory of developing mathematical reasoning.

---

Convergent vs Divergent Thinking

Again, many of the teachers I have discussed this activity with have shared their interest in finding the other possible versions of each shape.  However, what we would actually do with this activity seems to be quite different for each educator.  It seems like the decisions different teachers might be making here relate to their interest in students either having convergent thinking, or divergent thinking.  Let’s take a look at a few possible scenarios:

Teacher 1:

Before students start working on the activity, the teacher explains that their job is to find shapes that look exactly like the image in the picture at the top of the page.

Teacher 2:

Before students start working on the activity, the teacher tells the students exactly how many of each shape they found, then asks students to find them.

Teacher 3:

Before students start working on the activity, the teacher explains that their job is to find as many shapes as possible.  Then further explains that there might be ones that are not traditional looking.  Then, together with students, defines criteria for each shape they are about to look for.

Teacher 4:

Before students start working on the activity, the teacher explains that their job is to find as many shapes as possible.  As students are working, they challenge students to continue to think about other possibilities.

---

In the above scenarios, the teachers’ goals are quite different.  Teacher 1 expects their students to spend time looking at common versions of each shape, then spot them on the page.  Teacher 2’s aim is for students to be able to think deeper about what each shape really means, hoping that they are curious about where the rest of the shapes could possibly be leading their students to challenge themselves.  Teacher 3 believes that in order for students to be successful here, that they need to provide all of the potential pieces before their students get started.  Their goal in the end is for students to use the definitions they create together in the activity.  Finally, teacher 4’s goal is for students to access the mathematics before any terms or definitions are shared.  They believe that they can continue to push students to think by using effective questioning.  The development of reasoning is this teacher’s goal.

Looking back at these 4 teachers’ goals, I notice that 2 basic things differ:

1. How much scaffolding is provided; and
2. When scaffolding is provided

Teachers that provide lots of scaffolding prior to a problem typically aim for students to have convergent thinking.  They provide definitions and prompts, they model and tell, they hope that everyone will be able to get the same answers.

Teachers that withhold scaffolding and expect students to do more of the thinking along the way typically aim for divergent thinking.  That is, they hope that students will have different ideas in the hopes for students to share their thinking to create more thinking in others.

Whether you believe that convergent thinking or divergent thinking is best in math, I would really like you to consider how tasks that promote divergent thinking can actually help the group come to a consensus in the end.  If I were to provide this lesson to grade 2s, I would be aiming for students to be thinking as much as possible, to push students to continue to think outside-the-box as much as possible, then make sure that in my lesson close, that we ALL understood what makes a shape a shape.

---

I want to leave you with a few reflective questions:

• I provided you with a specific worksheet from a specific grade, however, I want you to now think about what you teach.  How much scaffolding do you provide?  Are you providing too much too soon?
• Do your lessons start off with convergent thinking or divergent thinking?  Why do you do this?  Is this because you believe it is best?
• How can you delay scaffolding and convergent thinking so that we are actually promoting our students to be actively thinking?  How can you make this a priority?
• What lesson or warm-ups or problems have you given that are examples of what we are talking about here?
• If we do remove some of the scaffolding will some of your students sit there not learning?  Is this a sign of them not understanding the math, or a sign of them used to being spoon-fed thinking?  What do WE need to get better at if we are to delay some of this scaffolding?

---

I encourage you to continue to think about what it means to help set up situations for your students to actively construct understanding:

• A Few Simple Beliefs
• Learning Goals, Success Criteria….. And Creativity?
• Never Forget the Closing of a Lesson!
• How do we meet the needs of so many unique students in a mixed-ability classroom?
• Differentiated Instruction: comparing 2 subjects

I’d love to continue the conversation.  Write a response, or send me a message on Twitter ( @markchubb3 ).

P.S.  I’m still not confident how many of each shape are actually here!